Cho ![]() và một điểm
và một điểm ![]() Có bao nhiêu điểm
Có bao nhiêu điểm ![]() thỏa mãn
thỏa mãn ![]()
Ta có . Suy ra tập hợp các điểm
thỏa mãn yêu cầu bài toán là đường tròn tâm
bán kính
.
Cho ![]() và một điểm
và một điểm ![]() Có bao nhiêu điểm
Có bao nhiêu điểm ![]() thỏa mãn
thỏa mãn ![]()
Ta có . Suy ra tập hợp các điểm
thỏa mãn yêu cầu bài toán là đường tròn tâm
bán kính
.
Trong hệ trục tọa độ ![]() , tọa độ của vectơ
, tọa độ của vectơ ![]() là
là
Ta có
Cho tam giác ABC. Gọi M là trung điểm BC và N là trung điểm AM. Đường thẳng BN cắt AC tại P. Khi đó ![]() thì giá trị của x là:
thì giá trị của x là:
Hình vẽ minh họa
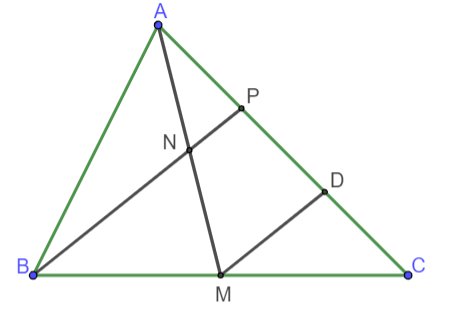
Kẻ . Do M là trung điểm BC
=> D là trung điểm CP (1).
Vì , mà N là trung điểm AM
=> P là trung điểm AD (2).
Từ (1), (2) ta suy ra .
=>
Ta có
=>
Ta có: (vì
ngược hướng)
=>
Trong mặt phẳng tọa độ ![]() , cho tam giác
, cho tam giác ![]() biết
biết ![]() . Tính độ dài đường trung tuyến kẻ từ đỉnh
. Tính độ dài đường trung tuyến kẻ từ đỉnh ![]() của tam giác
của tam giác ![]() ?
?
Gọi M là trung điểm của BC
Khi đó tọa độ của M là:
Suy ra độ dài đường trung tuyến kẻ từ đỉnh A hay độ dài đoạn AM là:
Vậy độ dài đường trung tuyến kẻ từ đỉnh A của tam giác ABC là .
Cho hai vectơ ![]() và
và ![]() đều khác vectơ
đều khác vectơ ![]() Tích vô hướng của
Tích vô hướng của ![]() và
và ![]() được xác định bằng công thức nào dưới đây?
được xác định bằng công thức nào dưới đây?
Cho hai vectơ và
đều khác vectơ
Tích vô hướng của
và
là một số, kí hiệu là
được xác định bởi công thức sau:
.
Mệnh đề nào sau đây đúng?
Vì vectơ - không cùng phương với mọi vectơ.
Cho ![]() Tìm tọa độ của vectơ
Tìm tọa độ của vectơ ![]()
Ta có
Cho tam giác ABC và điểm M thỏa mãn ![]() Xác định vị trí điểm M.
Xác định vị trí điểm M.
Giả sử G là trọng tâm tam giác ABC, khi đó ta có:
=> M là trọng tâm của tam giác ABC.
Cho hình bình hành ABCD tâm O. Mệnh đề nào sau đây là sai?
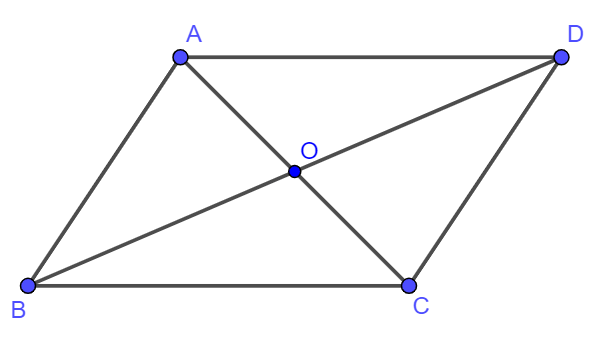
Ta có: (Sai).
Trong hệ tọa độ ![]() cho ba điểm
cho ba điểm ![]() và
và ![]() Tìm điểm
Tìm điểm ![]() thuộc trục hoành sao cho biểu thức
thuộc trục hoành sao cho biểu thức ![]() đạt giá trị nhỏ nhất.
đạt giá trị nhỏ nhất.
Ta có
Chọn điểm sao cho
Gọi , từ
ta có
Khi đó
Để nhỏ nhất
nhỏ nhất. Mà
thuộc trục hoành nên
nhỏ nhất khi
là hình chiếu vuông góc của
lên trục hoành
Trong mặt phẳng ![]() cho
cho ![]() . Tích vô hướng của 2 vectơ
. Tích vô hướng của 2 vectơ ![]() là:
là:
Ta có , suy ra
.
Cho tam giác ![]() cân tại
cân tại ![]() ,
, ![]() và
và ![]() . Tính
. Tính ![]() .
.
Ta có .
Cho tam giác ABC. Tập hợp các điểm M thỏa mãn ![]() là:
là:
Ta có: (I là trung điểm của BC)
=> Qũy tích điểm M là đường tròn đường kính IA.
Cho hình bình hành ABCD. Với mọi điểm M, ta có khẳng định nào sau đây:
Gọi O là giao điểm của AC và BD
=> OA OC, OB = OD
Ta có:
Cho hình vuông ![]() cạnh bằng
cạnh bằng ![]() . Tính độ dài véctơ
. Tính độ dài véctơ ![]() .
.
Hình vẽ minh họa:
Trong hệ tọa độ ![]() , cho tọa độ bốn điểm
, cho tọa độ bốn điểm ![]() ,
, ![]() . Chọn khẳng định đúng?
. Chọn khẳng định đúng?
Ta có: . Vậy
là hình bình hành.
Cho hình vuông ![]() cạnh
cạnh ![]() . Gọi
. Gọi ![]() là trung điểm của
là trung điểm của ![]() , lấy các điểm
, lấy các điểm ![]() lần lượt là các điểm thay đổi trên các cạnh
lần lượt là các điểm thay đổi trên các cạnh ![]() sao cho
sao cho ![]() . Tìm giá trị nhỏ nhất của biểu thức
. Tìm giá trị nhỏ nhất của biểu thức ![]() .
.
Hình vẽ minh họa
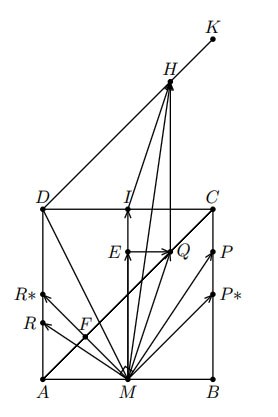
Đặt
Khi đó
Dấu bằng xảy ra khi và chỉ khi hay P, Q là trung điểm của BC, DA
Ta có:
Khi P ≡ P∗, R ≡ R∗, Q thay đổi trên AC, H sẽ thay đổi trên đoạn thẳng DK sao cho tam giác DCK vuông cân tại C.
Ta lại có:
Cho tam giác ![]() với
với ![]() là trung điểm
là trung điểm ![]() Mệnh đề nào sau đây đúng?
Mệnh đề nào sau đây đúng?
Xét đáp án Ta có
(theo quy tắc ba điểm).
Chọn đáp án này.
Cho hình bình hành ![]() . Đẳng thức nào sau đây đúng?
. Đẳng thức nào sau đây đúng?
Ta có:
sai do
.
sai do
.
sai do
.
đúng do
.
Cho tam giác ABC, có thể xác định được bao nhiêu vectơ khác ![]() có điểm đầu và điểm cuối là các đỉnh A, B, C?
có điểm đầu và điểm cuối là các đỉnh A, B, C?
Ta có các vectơ khác có điểm đầu và điểm cuối là các đỉnh tam giác ABC là:
Cho M là trung điểm AB, tìm đẳng thức sai
![]()
Ta có: .
Đáp án sai là .
Cho hình bình hành ![]() có
có ![]() là giao điểm của hai đường chéo. Gọi
là giao điểm của hai đường chéo. Gọi ![]() lần lượt là trung điểm của
lần lượt là trung điểm của ![]() . Đẳng thức nào sau đây sai?
. Đẳng thức nào sau đây sai?
Ta có lần lượt là đường trung bình của tam giác
và
.
là hình bình hành.
Cho tam giác ABC đều cạnh 2a. Đẳng thức nào sau đây là đúng?
Theo bài ra ta có:
Tam giác ABC đều cạnh 2a => AB = BC = AC = 2a
=>
Trong mặt phẳng tọa độ ![]() cho hai vectơ
cho hai vectơ ![]() và
và ![]() Tính tích vô hướng
Tính tích vô hướng ![]()
Ta có: và
Vậy
Cho hình vuông ![]() cạnh
cạnh ![]() Tính
Tính ![]()
Ta có
Cho ngũ giác ![]() . Từ các đỉnh của ngũ giác đã cho có thể lập được bao nhiêu vectơ có điểm cuối là điểm
. Từ các đỉnh của ngũ giác đã cho có thể lập được bao nhiêu vectơ có điểm cuối là điểm ![]() ?
?
Các vectơ có điểm cuối là điểm là
;
;
;
.
Cho tam giác ABC đều cạnh ![]() . Đường thẳng
. Đường thẳng ![]() qua
qua ![]() và song song với
và song song với ![]() , lấy điểm
, lấy điểm ![]() . Tính giá trị nhỏ nhất của
. Tính giá trị nhỏ nhất của ![]() khi
khi ![]() di động trên
di động trên ![]() .
.
Hình vẽ minh họa
Kẻ hình bình hành ACBD. Gọi I là trung điểm BD, khi đó, ta có
Ta có:
Dấu “=” xảy ra khi và chỉ khi M trùng với điểm H là hình chiếu vuông góc của điểm I trên đường thẳng .
Cho tứ giác ![]() . Có bao nhiêu vectơ khác vectơ - không có điểm đầu và cuối là các đỉnh của tứ giác?
. Có bao nhiêu vectơ khác vectơ - không có điểm đầu và cuối là các đỉnh của tứ giác?
Xét các vectơ có điểm là điểm đầu thì có các vectơ thỏa mãn bài toán là
có 3 vectơ.
Tương tự cho các điểm còn lại
Vậy chọn đáp án 12.
Cho tọa độ ba điểm ![]() . Tính
. Tính ![]() ?
?
Ta có:
Nếu ![]() là trọng tâm tam giác
là trọng tâm tam giác ![]() thì đẳng thức nào sau đây đúng?
thì đẳng thức nào sau đây đúng?
Gọi là trung điểm
.
Ta có .
Trong hệ tọa độ ![]() cho tam giác
cho tam giác ![]() có
có ![]() ,
, ![]() và
và ![]() thuộc trục
thuộc trục ![]() , trọng tâm
, trọng tâm ![]() của tam giác thuộc trục
của tam giác thuộc trục ![]() . Tìm tọa độ điểm
. Tìm tọa độ điểm ![]()
Vì thuộc trục
có hoành độ bằng
. Loại
.
Trọng tâm thuộc trục
có tung độ bằng
Xét các đáp án còn lại chỉ có đáp án
thỏa mãn
Trong mặt phẳng tọa độ Oxy, cho ![]() . Đâu là tọa độ của điểm A?
. Đâu là tọa độ của điểm A?
Ta có: O(0; 0)
Trong hệ tọa độ ![]() cho tam giác
cho tam giác ![]() có
có ![]() và trọng tâm
và trọng tâm ![]() . Tìm tọa độ đỉnh
. Tìm tọa độ đỉnh ![]() ?
?
Gọi
Vì là trọng tâm tam giác
nên
Tính tổng ![]() .
.
Ta có .
Điều kiện nào dưới đây là điều kiện cần và đủ để điểm ![]() là trung điểm của đoạn
là trung điểm của đoạn ![]() .
.
Điểm là trung điểm của đoạn
khi và chỉ khi
và ngược hướng.
Vậy .
Cho hình thang ![]() có đáy là
có đáy là ![]() và
và ![]() Gọi
Gọi ![]() và
và ![]() lần lượt là trung điểm của
lần lượt là trung điểm của ![]() và
và ![]() Khẳng định nào sau đây sai?
Khẳng định nào sau đây sai?
Vì lần lượt là trung điểm của
Dựa vào đáp án, ta có nhận xét sau:
đúng, vì
đúng, vì
đúng, vì
và
Suy ra
sai, vì theo phân tích ở đáp án trên. Chọn đáp án này.
Cho lục giác đều ![]() có tâm
có tâm ![]() Đẳng thức nào sau đây sai?
Đẳng thức nào sau đây sai?
Đẳng thức sai là
Cho tam giác ABC vuông tại A có AB = 3, AC = 4. Tính độ dài ![]()
Dựng hình bình hành tâm O như sau:

Ta có:
Vì tam giác AOB vuông tại A ta có:
Cho tam giác ![]() đều cạnh
đều cạnh ![]() Mệnh đề nào sau đây đúng?
Mệnh đề nào sau đây đúng?
Độ dài các cạnh của tam giác là thì độ dài các vectơ
.
Cho ![]() và một điểm C. Có bao nhiêu điểm D thỏa mãn
và một điểm C. Có bao nhiêu điểm D thỏa mãn ![]()
Có một và chỉ một điểm D thỏa mãn
