Lớp 12 Toán 12

Luyện tập Phương trình mũ và phương trình lôgarit (Khó)

Nhận biết Thông hiểu Vận dụng Vận dụng cao
  • Bài kiểm tra này bao gồm 20 câu
  • Điểm số bài kiểm tra: 20 điểm
  • Xem lại kỹ lý thuyết trước khi làm bài
  • Chuẩn bị giấy và bút để nháp trước khi bắt đầu
Bắt đầu làm bài
00:00:00
  • Câu 1: Thông hiểu
    Tìm nghiệm nguyên nhỏ nhất

    Nghiệm nguyên nhỏ nhất của phương trình - {\log _{\sqrt 3 }}\left( {x - 2} ight).{\log _5}x = 2{\log _3}\left( {x - 2} ight) là?

    3 || ba || Ba

    Đáp án là:

    Nghiệm nguyên nhỏ nhất của phương trình - {\log _{\sqrt 3 }}\left( {x - 2} ight).{\log _5}x = 2{\log _3}\left( {x - 2} ight) là?

    3 || ba || Ba

    Điều kiện: x>2

    Ta có: - {\log _{\sqrt 3 }}\left( {x - 2} ight).{\log _5}x = 2{\log _3}\left( {x - 2} ight)

    \Leftrightarrow - 2{\log _3}\left( {x - 2} ight).{\log _5}x = 2{\log _3}\left( {x - 2} ight)

    \Leftrightarrow \left[ \begin{gathered} {\log _3}\left( {x - 2} ight) = 0 \hfill \\ {\log _5}x = - 1 \hfill \\ \end{gathered} ight. 

    \Leftrightarrow \left[ \begin{gathered} {\log _3}\left( {x - 2} ight) = 0 \hfill \\ {\log _5}x = - 1 \hfill \\ \end{gathered} ight. \Leftrightarrow \left[ \begin{gathered} x = 3 \hfill \\ x = \frac{1}{5} \hfill \\ \end{gathered} ight.

    So điều kiện suy ra phương trình có nghiệm x=3.

  • Câu 2: Vận dụng cao
    Khẳng định đúng?

    Biết phương trình \frac{1}{{{{\log }_2}x}} - \frac{1}{2}{\log _2}x + \frac{7}{6} = 0 có hai nghiệm x_1, x_2. Khẳng định nào sau đây là đúng?

    Hướng dẫn:

    Điều kiện: \left\{ \begin{gathered} x > 0 \hfill \\ {\log _2}x e 0 \hfill \\ \end{gathered} ight. \Leftrightarrow \left\{ \begin{gathered} x > 0 \hfill \\ x e 1 \hfill \\ \end{gathered} ight..

    Đặt t = {\log _2}x. Phương trình đã cho trở thành 3{t^2} - 7t - 6 = 0.

    \Leftrightarrow \left[ \begin{gathered} t = 3 \hfill \\ t = - \frac{2}{3} \hfill \\ \end{gathered} ight.\Leftrightarrow \left[ \begin{gathered} {\log _2}x = 3 \hfill \\ {\log _2}x = - \frac{2}{3} \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left[ \begin{gathered} x = {2^3} = 9 \hfill \\ x = {2^{ - \frac{2}{3}}} = \frac{1}{{\sqrt[3]{4}}} \hfill \\ \end{gathered} ight. (thỏa mãn điều kiện)

    Vậy tập nghiệm của phương trình đã cho là S = \left\{ {8;\frac{1}{{\sqrt[3]{4}}}} ight\} \Rightarrow x_1^3 + x_2^3 = \frac{{2049}}{4}.

  • Câu 3: Vận dụng cao
    Tìm m để có 2 nghiệm trái dấu

    Với giá trị của tham số m thì phương trình \left( {m + 1} ight){16^x} - 2\left( {2m - 3} ight){4^x} + 6m + 5 = 0 có hai nghiệm trái dấu?

    Hướng dẫn:

    Đặt {4^x} = t > 0

    Phương trình đã cho trở thành: \underbrace {\left( {m + 1} ight){t^2} - 2\left( {2m - 3} ight)t + 6m + 5}_{f\left( t ight)} = 0

    Yêu cầu bài toán \Leftrightarrow \left( * ight) có hai nghiệm {t_1},{\text{ }}{t_2} thỏa mãn 0 < {t_1} < 1 < {t_2}.

    \ \Leftrightarrow \left\{ \begin{gathered} m + 1 e 0 \hfill \\ \left( {m + 1} ight)f\left( 1 ight) < 0 \hfill \\ \left( {m + 1} ight)\left( {6m + 5} ight) > 0 \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left\{ \begin{gathered} m + 1 e 0 \hfill \\ \left( {m + 1} ight)\left( {3m + 12} ight) < 0 \hfill \\ \left( {m + 1} ight)\left( {6m + 5} ight) > 0 \hfill \\ \end{gathered} ight. \Leftrightarrow - 4 < m < - 1

  • Câu 4: Vận dụng cao
    Tổng 2 nghiệm PT mũ

    Gọi x_1, x_2 là hai nghiệm của phương trình {2^{{x^2} + 4}} = {2^{2\left( {{x^2} + 1} ight)}} + \sqrt {{2^{2\left( {{x^2} + 2} ight)}} - {2^{{x^2} + 3}} + 1}.  Khi đó, tổng hai nghiệm bằng?

    Hướng dẫn:

     Ta có: {2^{{x^2} + 4}} = {2^{2\left( {{x^2} + 1} ight)}} + \sqrt {{2^{2\left( {{x^2} + 2} ight)}} - {2^{{x^2} + 3}} + 1}

    \Leftrightarrow {8.2^{{x^2} + 1}} = {2^{2\left( {{x^2} + 1} ight)}} + \sqrt {{{4.2}^{2\left( {{x^2} + 1} ight)}} - {{4.2}^{{x^2} + 1}} + 1}

    Đặt t = {2^{{x^2} + 1}}\left( {t \geqslant 2} ight), phương trình trên tương đương với:

    8t = {t^2} + \sqrt {4{t^2} - 4t + 1} \Leftrightarrow {t^2} - 6t - 1 = 0 \Leftrightarrow t = 3 + \sqrt {10} (vì t \geq 2).

    Từ đó suy ra {2^{{x^2} + 1}} = 3 + \sqrt {10} \Leftrightarrow \left[ \begin{gathered} {x_1} = \sqrt {{{\log }_2}\frac{{3 + \sqrt {10} }}{2}} \hfill \\ {x_2} = - \sqrt {{{\log }_2}\frac{{3 + \sqrt {10} }}{2}} \hfill \\ \end{gathered} ight.

     

    Vậy tổng hai nghiệm bằng 0.

  • Câu 5: Vận dụng cao
    Tìm m thỏa mãn

    Với giá trị nào của tham số m thì phương trình {4^x} - m{.2^{x + 1}} + 2m = 0 có hai nghiệm {x_1},{\text{ }}{x_2} thoả mãn {x_1} + {x_2} = 3 ?

    Hướng dẫn:

     Ta có: {4^x} - m{.2^{x + 1}} + 2m = 0 \Leftrightarrow {\left( {{2^x}} ight)^2} - 2m{.2^x} + 2m = 0{\text{ }}\left( * ight)

    Phương trình (*) là phương trình bậc hai ẩn 2^x có: \Delta ' = {\left( { - m} ight)^2} - 2m = {m^2} - 2m.

    Phương trình (*) có nghiệm \Leftrightarrow {m^2} - 2m \geqslant 0 \Leftrightarrow m\left( {m - 2} ight) \geqslant 0 \Leftrightarrow \left[ \begin{gathered} m \geqslant 2 \hfill \\ m \leqslant 0 \hfill \\ \end{gathered} ight.

    Áp dụng định lý Vi-ét ta có: {2^{{x_1}}}{.2^{{x_2}}} = 2m \Leftrightarrow {2^{{x_1} + {x_2}}} = 2m

    Do đó {x_1} + {x_2} = 3 \Leftrightarrow {2^3} = 2m \Leftrightarrow m = 4.

    Thử lại ta được m=4 thỏa mãn.

  • Câu 6: Thông hiểu
    Tìm tập nghiệm của PT logarit

    Phương trình \log _2^2x - 4{\log _2}x + 3 = 0 có tập nghiệm là?

    Hướng dẫn:

    Điều kiện: x > 0

    \log _2^2x - 4{\log _2}x + 3 = 0 \Leftrightarrow \left[ \begin{gathered} {\log _2}x = 1 \hfill \\ {\log _2}x = 3 \hfill \\ \end{gathered} ight. \Leftrightarrow \left[ \begin{gathered} x = 2 \hfill \\ x = 8 \hfill \\ \end{gathered} ight.

    Vậy PT có tập nghiệm là S={8;2}.

  • Câu 7: Vận dụng
    Đếm số nghiệm thực

    Phương trình {\left( {\sqrt 3 - \sqrt 2 } ight)^x} + {\left( {\sqrt 3 + \sqrt 2 } ight)^x} = {\left( {\sqrt {10} } ight)^x} có tất cả bao nhiêu nghiệm thực ?

    Hướng dẫn:

     Ta có: {\left( {\sqrt 3 - \sqrt 2 } ight)^x} + {\left( {\sqrt 3 + \sqrt 2 } ight)^x} = {\left( {\sqrt {10} } ight)^x}\Leftrightarrow {\left( {\frac{{\sqrt 3 - \sqrt 2 }}{{\sqrt {10} }}} ight)^x} + {\left( {\frac{{\sqrt 3 + \sqrt 2 }}{{\sqrt {10} }}} ight)^x} = 1

    Xét hàm số f\left( x ight) = {\left( {\frac{{\sqrt 3 - \sqrt 2 }}{{\sqrt {10} }}} ight)^x} + {\left( {\frac{{\sqrt 3 + \sqrt 2 }}{{\sqrt {10} }}} ight)^x}

    Ta có: f\left( 2 ight) = 1

    Hàm số f (x) nghịch biến trên R do các cơ số \frac{{\sqrt 3 - \sqrt 2 }}{{\sqrt {10} }} < 1;\frac{{\sqrt 3 + \sqrt 2 }}{{\sqrt {10} }} < 1.

    Vậy phương trình có nghiệm duy nhất là x=2.

  • Câu 8: Thông hiểu
    Điều kiện xác định

    Điều kiện xác định của phương trình \log ({x^2} - 6x + 7) + x - 5 = \log (x - 3) là:

    Hướng dẫn:

    Điều kiện phương trình xác định:  

    \left\{ \begin{gathered} {x^2} - 6{\text{x + 7}} > 0 \hfill \\ x - 3 > 0 \hfill \\ \end{gathered} ight. \Leftrightarrow \left\{ \begin{gathered} \left[ \begin{gathered} x > 3 + \sqrt 2 \hfill \\ x < 3 - \sqrt 2 \hfill \\ \end{gathered} ight. \hfill \\ x > 3 \hfill \\ \end{gathered} ight. \Leftrightarrow x > 3 + \sqrt 2

  • Câu 9: Thông hiểu
    Tính tích

    Gọi x_1, x_2là nghiệm của phương trình {\log _x}2 - {\log _{16}}x = 0. Khi đó tích x_1.x_2 bằng:

    1 || x1.x2=1

    Đáp án là:

    Gọi x_1, x_2là nghiệm của phương trình {\log _x}2 - {\log _{16}}x = 0. Khi đó tích x_1.x_2 bằng:

    1 || x1.x2=1

    Điều kiện: 0 < x e 1

    PT \Leftrightarrow {\log _x}2 - {\log _{16}}x = 0 \Leftrightarrow {\log _x}2 - {\log _{{2^4}}}x = 0 \Leftrightarrow {\log _x}2 - \frac{1}{4}{\log _2}x = 0

    \Leftrightarrow {\log _x}2 - \frac{1}{{4{{\log }_x}2}} = 0 \Leftrightarrow \frac{{4{{({{\log }_x}2)}^2} - 1}}{{4{{\log }_x}2}} = 0 \Leftrightarrow 4{({\log _x}2)^2} - 1 = 0

    \Leftrightarrow {({\log _x}2)^2} = \frac{1}{4} \Leftrightarrow \left[ \begin{gathered} {\log _x}2 = \frac{1}{2} \hfill \\ {\log _x}2 = - \frac{1}{2} \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left[ \begin{gathered} 2 = {x^{\frac{1}{2}}} \hfill \\ 2 = {x^{ - \frac{1}{2}}} \hfill \\ \end{gathered} ight. \Leftrightarrow \left[ \begin{gathered} {x_1} = 4 \hfill \\ {x_2} = \frac{1}{4} \hfill \\ \end{gathered} ight.

    Vậy {x_1}.{x_2} = 4.\frac{1}{4} = 1.

  • Câu 10: Vận dụng cao
    Tính tích các nghiệm

    Tích các nghiệm của phương trình {\log _2}x.{\log _4}x.{\log _8}x.{\log _{16}}x = \frac{{81}}{{24}}  là:

    Hướng dẫn:

    Điều kiện: x >0

    Ta có: {\log _2}x.{\log _4}x.{\log _8}x.{\log _{16}}x = \frac{{81}}{{24}} \Leftrightarrow \left( {{{\log }_2}x} ight)\left( {\frac{1}{2}{{\log }_2}x} ight)\left( {\frac{1}{3}{{\log }_2}x} ight)\left( {\frac{1}{4}{{\log }_2}x} ight) = \frac{{81}}{{24}}

    \Leftrightarrow \log _2^4 = 81 \Leftrightarrow {\log _2}x = \pm 3 \Leftrightarrow x = 8  hoặc x = \frac{1}{8}. (thỏa mãn điều kiện)

    Vậy tập nghiệm của phương trình đã cho là S = \left\{ {\frac{1}{8};8} ight\} \Rightarrow {x_1}.{x_2} = 1.

  • Câu 11: Thông hiểu
    Phương trình trở thành

    Nếu đặt t = {\log _2}x thì phương trình \frac{1}{{5 - {{\log }_2}x}} + \frac{2}{{1 + {{\log }_2}x}} = 1 trở thành phương trình nào?

    Hướng dẫn:

    Đặt t = {\log _2}x

    PT \Leftrightarrow \frac{1}{{5 - t}} + \frac{2}{{1 + t}} = 1 \Leftrightarrow \frac{{1 + t + 2(5 - t)}}{{(5 - t)(1 + t)}} = 1

    \Leftrightarrow 1 + t + 2(5 - t) = (5 - t)(1 + t)

    \Leftrightarrow 11 - t = 5 + 4t - {t^2} \Leftrightarrow {t^2} - 5t + 6 = 0.

  • Câu 12: Vận dụng cao
    Tìm tất cả các giá trị thực của m

    Tìm tất cả các giá trị thực của tham số m để phương trình \log _3^2x + \sqrt {\log _3^2x + 1} - 2m - 1 = 0 có ít nhất một nghiệm thuộc đoạn \left[ {1;{3^{\sqrt 3 }}} ight]?

    Hướng dẫn:

     Với x \in \left[ {1;{3^{\sqrt 3 }}} ight] hay 1 \leqslant x \leqslant {3^{\sqrt 3 }} \Rightarrow \sqrt {\log _3^21 + 1} \leqslant \sqrt {\log _3^2x + 1} \leqslant \sqrt {\log _3^2{3^{\sqrt 3 }} + 1} hay 1 \leqslant t \leqslant 2.

    Khi đó bài toán được phát biểu lại là: “Tìm m để phương trình có ít nhất một nghiệm thuộc đoạn [1;2]”.

    Ta có PT \Leftrightarrow 2m = {t^2} + t + 2

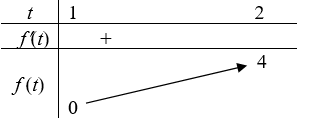
    Xét hàm số f(t) = {t^2} + t - 2,{\text{ }}\forall t \in \left[ {1;2} ight],{\text{ }}f'(t) = 2t + 1 > 0,{\text{ }}\forall t \in \left[ {1;2} ight]

    Suy ra hàm số đồng biến trên [1;2].

    Khi đó phương trình có nghiệm khi 0 \leqslant 2m \leqslant 4 \Leftrightarrow 0 \leqslant m \leqslant 2.

    Vậy 0 \leqslant m \leqslant 2  là các giá trị của m cần tìm.

  • Câu 13: Vận dụng
    Chọn phát biểu đúng

    Phương trình {2^{x - 3}} = {3^{{x^2} - 5x + 6}} có hai nghiệm x_1, x_2 trong đó x_1 < x_2, hãy chọn phát biểu đúng?

    Hướng dẫn:

     Logarit hóa hai vế của phương trình (theo cơ số 2) ta được:

    {2^{x - 3}} = {3^{{x^2} - 5x + 6}} \Leftrightarrow {\log _2}{2^{x - 3}} = {\log _2}{3^{{x^2} - 5x + 6}}

    \Leftrightarrow \left( {x - 3} ight){\log _2}2 = \left( {{x^2} - 5x + 6} ight){\log _2}3

    \Leftrightarrow \left( {x - 3} ight) - \left( {x - 2} ight)\left( {x - 3} ight){\log _2}3 = 0

    \Leftrightarrow \left( {x - 3} ight).\left[ {1 - \left( {x - 2} ight){{\log }_2}3} ight] = 0 \Leftrightarrow \left[ \begin{gathered} x - 3 = 0 \hfill \\ 1 - \left( {x - 2} ight){\log _2}3 \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left[ \begin{gathered} x = 3 \hfill \\ \left( {x - 2} ight){\log _2}3 = 1 \hfill \\ \end{gathered} ight. \Leftrightarrow \left[ \begin{gathered} x = 3 \hfill \\ x - 2 = \frac{1}{{{{\log }_2}3}} \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left[ \begin{gathered} x = 3 \hfill \\ x = {\log _3}2 + 2 \hfill \\ \end{gathered} ight. \Leftrightarrow \left[ \begin{gathered} x = 3 \hfill \\ x = {\log _3}2 + {\log _3}9 \hfill \\ \end{gathered} ight. \Leftrightarrow \left[ \begin{gathered} x = 3 \hfill \\ x = {\log _3}18 \hfill \\ \end{gathered} ight.

  • Câu 14: Nhận biết
    Số nghiệm của PT logarit

    Phương trình \log _2^2(x + 1) - 6{\log _2}\sqrt {x + 1} + 2 = 0 có số nghiệm là:

    2 || hai || 2 nghiệm || Hai nghiệm

    Đáp án là:

    Phương trình \log _2^2(x + 1) - 6{\log _2}\sqrt {x + 1} + 2 = 0 có số nghiệm là:

    2 || hai || 2 nghiệm || Hai nghiệm

     PT\Leftrightarrow \left\{ \begin{gathered} x + 1 > 0 \hfill \\ {\log ^2}_2(x + 1) - 3{\log _2}(x + 1) + 2 = 0 \hfill \\ \end{gathered} ight. \Leftrightarrow \left\{ \begin{gathered} x > - 1 \hfill \\ \left[ \begin{gathered} {\log _2}(x + 1) = 1 \hfill \\ {\log _2}(x + 1) = 2 \hfill \\ \end{gathered} ight. \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left\{ \begin{gathered} x > - 1 \hfill \\ \left[ \begin{gathered} x = 1 \hfill \\ x = 3 \hfill \\ \end{gathered} ight. \hfill \\ \end{gathered} ight. \Leftrightarrow \left[ \begin{gathered} x = 1 \hfill \\ x = 3 \hfill \\ \end{gathered} ight.

    Vậy PT có 2 nghiệm.

  • Câu 15: Nhận biết
    Đếm số nghiệm

    Số nghiệm của phương trình {\log _2}x.{\log _3}(2x - 1) = 2{\log _2}x là:

    2 || hai nghiệm || Hai nghiệm || 2 nghiệm

    Đáp án là:

    Số nghiệm của phương trình {\log _2}x.{\log _3}(2x - 1) = 2{\log _2}x là:

    2 || hai nghiệm || Hai nghiệm || 2 nghiệm

     PT \Leftrightarrow \left\{ \begin{gathered} x > 0 \hfill \\ 2x - 1 > 0 \hfill \\ {\log _2}x.{\log _3}(2x - 1) = 2{\log _2}x \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left\{ \begin{gathered} x > \frac{1}{2} \hfill \\ {\log _2}x\left[ {{{\log }_3}(2x - 1) - 2} ight] = 0 \hfill \\ \end{gathered} ight. 

    \Leftrightarrow \left\{ \begin{gathered} x > \frac{1}{2} \hfill \\ \left[ \begin{gathered} {\log _2}x = 0 \hfill \\ {\log _3}(2x - 1) = 2 \hfill \\ \end{gathered} ight. \hfill \\ \end{gathered} ight. \Leftrightarrow \left\{ \begin{gathered} x > \frac{1}{2} \hfill \\ \left[ \begin{gathered} x = 1 \hfill \\ x = 5 \hfill \\ \end{gathered} ight. \hfill \\ \end{gathered} ight. \Leftrightarrow \left[ \begin{gathered} x = 1 \hfill \\ x = 5 \hfill \\ \end{gathered} ight.

    Vậy PT có hai nghiệm.

  • Câu 16: Vận dụng
    Tính tích 2 nghiệm

    Gọi x_1, x_2 là 2 nghiệm của phương trình \frac{1}{{4 + {{\log }_2}x}} + \frac{2}{{2 - {{\log }_2}x}} = 1. Khi đó x_1.x_2 bằng:

    Hướng dẫn:

     Điều kiện: \left\{ \begin{gathered} x > 0 \hfill \\ x e 4 \hfill \\ x e \frac{1}{{16}} \hfill \\ \end{gathered} ight..

    Đặt t = {\log _2}x ,điều kiện \left\{ \begin{gathered} t e - 4 \hfill \\ t e 2 \hfill \\ \end{gathered} ight.. Khi đó phương trình trở thành:

    \frac{1}{{4 + t}} + \frac{2}{{2 - t}} = 1 \Leftrightarrow {t^2} + 3t + 2 = 0 \Leftrightarrow \left[ \begin{gathered} t = - 1 \hfill \\ t = - 2 \hfill \\ \end{gathered} ight. \Rightarrow \left[ \begin{gathered} x = \frac{1}{2} \hfill \\ x = \frac{1}{4} \hfill \\ \end{gathered} ight.

    Vậy {x_1}.{x_2} = \frac{1}{8}.

  • Câu 17: Nhận biết
    Giải PT Logarit

    Phương trình {\log _2}(x + 3) + {\log _2}(x - 1) = {\log _2}5 có nghiệm là:

    2 || hai || x=2 || Hai

    Đáp án là:

    Phương trình {\log _2}(x + 3) + {\log _2}(x - 1) = {\log _2}5 có nghiệm là:

    2 || hai || x=2 || Hai

     PT \Leftrightarrow \left\{ \begin{gathered} x - 1 > 0 \hfill \\ (x + 3)(x - 1) = 5 \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left\{ \begin{gathered} x > 1 \hfill \\ {x^2} + 2x - 8 = 0 \hfill \\ \end{gathered} ight. \Leftrightarrow \left\{ \begin{gathered} x > 1 \hfill \\ \left[ \begin{gathered} x = - 8 \hfill \\ x = 2 \hfill \\ \end{gathered} ight. \hfill \\ \end{gathered} ight. \Rightarrow x = 2

  • Câu 18: Vận dụng
    Tìm họ nghiệm

    Phương trình {9^{{{\sin }^2}x}} + {9^{{{\cos }^2}x}} = 6 có họ nghiệm là ?

    Hướng dẫn:

     Ta có: {9^{{{\sin }^2}x}} + {9^{{{\cos }^2}x}} = 6

    \Leftrightarrow {9^{1 - {{\cos }^2}x}} + {9^{{{\cos }^2}x}} = 6 \Leftrightarrow \frac{9}{{{9^{{{\cos }^2}x}}}} + {9^{{{\cos }^2}x}} - 6 = 0{\text{ }}\left( * ight)

    Đặt t = {9^{{{\cos }^2}x}},{\text{ }}\left( {1 \leqslant t \leqslant 9} ight).

    Khi đó: \left( * ight) \Leftrightarrow \frac{9}{t} + t - 6 = 0 \Leftrightarrow {t^2} - 6t + 9 = 0 \Leftrightarrow t = 3.

    Với t = 3 \Rightarrow {9^{{{\cos }^2}x}} = 3 \Leftrightarrow {3^{2{{\cos }^2}x}} = {3^1} \Leftrightarrow 2{\cos ^2}x - 1 = 0

    \Leftrightarrow \cos 2x = 0 \Leftrightarrow \boxed{x = \frac{\pi }{4} + \frac{{k\pi }}{2}},{\text{ }}\left( {k \in \mathbb{Z}} ight).

  • Câu 19: Nhận biết
    Tìm tập nghiệm PT Logarit

    Phương trình \log _2^{}x + {\log _2}(x - 1) = 1 có tập nghiệm là:

    {2} || T={2}

    Đáp án là:

    Phương trình \log _2^{}x + {\log _2}(x - 1) = 1 có tập nghiệm là:

    {2} || T={2}

     PT \Leftrightarrow \left\{ \begin{gathered} x > 0 \hfill \\ x - 1 > 0 \hfill \\ {\log _2}\left[ {x(x - 1)} ight] = 1 \hfill \\ \end{gathered} ight. \Leftrightarrow \left\{ \begin{gathered} x > 1 \hfill \\ {x^2} - x - 2 = 0 \hfill \\ \end{gathered} ight.

    \Leftrightarrow \left\{ \begin{gathered} x > 1 \hfill \\ \left[ \begin{gathered} x = - 1 \hfill \\ x = 2 \hfill \\ \end{gathered} ight. \hfill \\ \end{gathered} ight. \Leftrightarrow x = 2.

  • Câu 20: Nhận biết
    Tìm nghiệm của PT

    Phương trình {\log _2}(3x - 2) = 2 có nghiệm là: 

    x=2 || 2 || hai

    Đáp án là:

    Phương trình {\log _2}(3x - 2) = 2 có nghiệm là: 

    x=2 || 2 || hai

     PT \Leftrightarrow \left\{ \begin{gathered} 3x - 2 > 0 \hfill \\ 3x - 2 = 4 \hfill \\ \end{gathered} ight. \Leftrightarrow \left\{ \begin{gathered} x > \frac{3}{2} \hfill \\ x = 2 \hfill \\ \end{gathered} ight. \Leftrightarrow x = 2.

0/20
20 câu:
Kiểm tra Kiểm tra lại Bỏ qua Nộp bài

Chúc mừng Bạn đã hoàn thành bài!

Kết quả làm bài:
  • Nhận biết (25%):
    2/3
  • Thông hiểu (25%):
    2/3
  • Vận dụng (20%):
    2/3
  • Vận dụng cao (30%):
    2/3
  • Thời gian làm bài: 00:00:00
  • Số câu làm đúng: 0
  • Số câu làm sai: 0
  • Điểm số: 0
Làm lại
  • 1 lượt xem

Nội dung cùng chủ đề

Sắp xếp theo
🖼️
Xóa Gửi bình luận

Toán 12

Đăng nhập